If you happen to be viewing the article Introduction to Median of a Triangle? on the website Math Hello Kitty, there are a couple of convenient ways for you to navigate through the content. You have the option to simply scroll down and leisurely read each section at your own pace. Alternatively, if you’re in a rush or looking for specific information, you can swiftly click on the table of contents provided. This will instantly direct you to the exact section that contains the information you need most urgently.
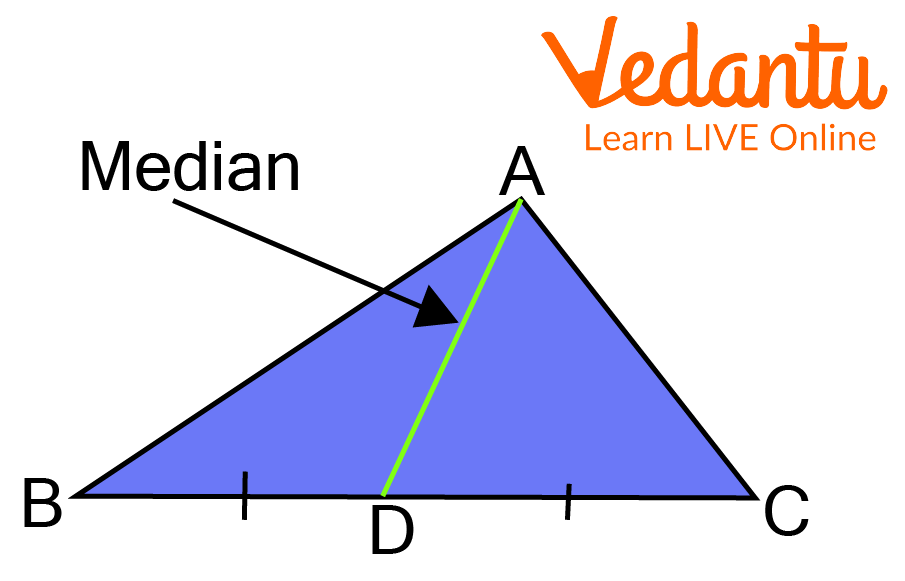
A line segment joining a triangle vertex to the middle of the other side, bisecting that side, is referred to as the median of a triangle in geometry. There are three medians in every triangle, one from each vertex.
At the centroid of the triangle, these medians cross. The median of a triangle is a line segment that connects a vertex to the midpoint of the side that is opposite to that vertex. The median (AD) in the example below divides BC into two equal portions, resulting in BD = DC. This answers, what is a median in geometry?
Median of a Triangle
Contents
Properties of Median of a Triangle
A few properties of median of a triangle are listed below:
-
A line segment from a triangle’s vertex to the middle of its opposite side is said to be the triangle’s median.
-
It divides the opposing side into two equal portions by cutting it in half.
-
A triangle is further divided into two triangles with the same area by its median.
-
Any triangle’s three medians meet at a single point, regardless of its size or shape.
-
Three medians, one from each vertex, exist for each triangle. The triangle’s centroid is formed by the intersection of three medians.
-
The centroid is the point of concurrency of medians of the triangle.
The Altitude of a Triangle
A line segment making a straight angle (90°) from a triangle’s vertex to its opposite side is considered the triangle’s altitude. Depending on the type of triangle, an altitude may be inside or outside the triangle. Each triangle has three altitudes, one from each vertex, which all come together at the triangle’s orthocenter.
How to Find the Median of a Triangle
The median formula geometry is given as follows.
The first median of a triangle formula is calculated using the median of a triangle theorem, where the triangle’s median is $m_{a}$, its sides are $mathrm{a}, mathrm{b}$, and $mathrm{c}$, and the median is established on the side ‘ $a$ ‘.
$m_{a}=sqrt{dfrac{2 b^{2}+2 c^{2}-a^{2}}{4}}$
The second median of a triangle is calculated using the median of a triangle theorem, where the triangle’s median is $m_{b}$, its sides are a, b, and $c$, and the median is established on the side ‘ $b$ ‘.
$m_{h}=sqrt{dfrac{2 a^{2}+2 c^{2}-b^{2}}{4}}$
The third median of a triangle is calculated using the following formula, where the triangle’s median is $m_{c}$, its sides are $mathrm{a}, mathrm{b}$, and $mathrm{c}$, and the median is established on side ‘ $c$ ‘.
$m_{c}=sqrt{dfrac{2 a^{2}+2 b^{2}-c^{2}}{4}}$
Solved Examples
Q1. Calculate the length of the median of the triangle PQR, whose sides are PQ = 3, PR = 4, and QR = 5 units. The median created on the side QR is given as PM.

Example to Find the Median
Ans: Here, we have to find a PM. So, we have to use the median of the triangle formula i.e.
$P M=sqrt{dfrac{2 P Q^{2}+2 P R^{2}-Q R^{2}}{4}}$
$P M=sqrt{dfrac{2(3)^{2}+2(4)^{2}-(5)^{2}}{4}}$
where $P Q=3, P R=4, Q R=5$
$PM=sqrt{dfrac{18+32-25}{4}}=sqrt{dfrac{50-25}{4}}=sqrt{dfrac{25}{4}}=dfrac{5}{2}=2.5 text { units. }$
Q2. Determine the length of the median of the triangle ABC, whose sides are given as AB = 10, BC = 8, and AC = 13 units, respectively. The median created on side BC is given as AD where D is the median.
Ans: Here, we have to find AD. So, we have to use the median of the triangle formula i.e.
$A D=sqrt{dfrac{2 A B^{2}+2 A C^{2}-B C^{2}}{4}}$
$A D=sqrt{dfrac{2(10)^{2}+2(13)^{2}-(8)^{2}}{4}}$
where $A B=10, B C=8, A C=13$
$A D=sqrt{dfrac{200+338-64}{4}}=sqrt{dfrac{474}{4}}=10.88$ units.
Q3. Triangles have a 28 cm, 45 cm, and 53 cm median. What is the triangle’s area?
Ans: We have given the Pythagoras triplet i.e. 28cm, 45cm, 53cm.
Thus, the area of a triangle is equal to $dfrac{4}{3}$ which is multiplied by the area of the triangle formed by the median.
The area of a triangle is $dfrac{4}{3} times dfrac{1}{2} times 28 times 45=840 mathrm{~cm}^{2}$.
Q4. For the given triangle ABC, G is the centroid and BC = 10 units. Determine the length of BD.
Ans: Given,
$angle mathrm{GDC}=90$
$Rightarrow mathrm{GD}=18 times dfrac{1}{3}=6$
$Rightarrow mathrm{BG}=15 times dfrac{2}{3}=10$
In BGD,
Using Pythagoras theorem,
$Rightarrow B G^{2}=G D^{2}+B D^{2}$
$Rightarrow B D^{2}=100-36=64$
$Rightarrow B D=8$
$Rightarrow B C=2 B D=2 times 8=16$
Area of $Delta mathrm{BGD}=dfrac{1}{2} times mathrm{GD} times mathrm{BD}$
$=dfrac{1}{2} times 8 times 6=24$ sq.units.
Q5. Two equilateral triangles of side 4 cm each but labelled as △ABC and △LHN are not congruent. True or False?
Ans: Two equilateral triangles with equal sides are always congruent no matter how they are labelled. So the statement is False.
Practice Questions
Q1. Write the formula for the median of a triangle if it is given that there is a triangle MNO and MP is the median?
Ans: $M P=sqrt{dfrac{2 M N^{2}+2 M O^{2}-N O^{2}}{4}}$
Q2. Find the length of median AD if we have the coordinates of triangle ABC as A(1,0), B(0,1), C(1,1)
Ans: $dfrac{sqrt{5}}{2}$
Q3. Given statement is true or false i.e. point where median meets opposite sides which is the midpoint of that line.
Ans: True
Q4. For any triangle, the centroid is the point of concurrency of the ____________ of the triangle
Ans: Medians
Q5. How many medians do a triangle have?
Ans: Every triangle has 3 medians
Summary
In geometry, the median of a triangle is the line segment that connects one vertex to the middle of the other side, dividing it in half. Each vertex of a triangle has the same number of medians, which all cross at the triangle’s centroid. A median cuts any angle at an angle at the vertex of an isosceles or equilateral triangle whose two adjacent sides are of equal length. Since the corresponding elements of congruent triangles are congruent, the medians of congruent triangles are equal if the two triangles are congruent. Students can become well-versed only by practising many examples of the median of a triangle sum.
Thank you so much for taking the time to read the article titled Introduction to Median of a Triangle written by Math Hello Kitty. Your support means a lot to us! We are glad that you found this article useful. If you have any feedback or thoughts, we would love to hear from you. Don’t forget to leave a comment and review on our website to help introduce it to others. Once again, we sincerely appreciate your support and thank you for being a valued reader!
Source: Math Hello Kitty
Categories: Math